INFO DS 1 ES 17 /12/11

          DS     1ES                                 Samedi 17 décembre 2011       2 h

            Calculatrice autorisée .

     EXERCICE 1

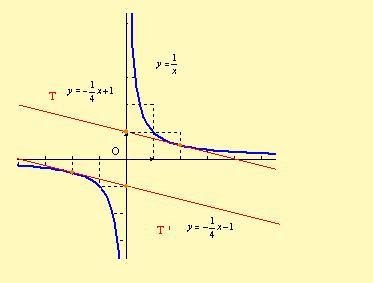
                  Soit l’hyperbole  H : y = 1 / x   .

1.        Tracer la courbe ( H ) de la fonction  h : x → 1 / x dans

            un repère orthonormal .

2.        Trouver l’équation  réduite de la tangente T à ( H ) au point d’abscisse 2.

3.        Trouver  un second point de la courbe ( H )  où la tangente  ( T ’ )

           est parallèle  à T .

------------------------------------------------------------------------------------------------------------------

   Réponse :

              1. Courbe ( H ).     C'est une hyperbole.

              2. Recherche de l'équation réduite de la tangente ( T ).

                  La fonction   h : x → 1 / x est définie et dérivable dans IR*.

                 On a :   h ' : x → - 1 / x²    

                 Donc       h' ( 2 ) = - 1 /  4       et    h ( 2 ) = 1/ 2

                 On a  :

                          T : y = h' ( 2 ) x + h ( 2 ) - 2 h '( 2 )

                 c-à-d    T : y = - 1 / 4  x + 1 / 2 - 2 ( - 1/ 4 )

                 c-à-d    T :  y = - 1 / 4 x + 1 / 2 + 1 / 2

              . Conclusion :     T : y = - 1/ 4  x   + 1

              3. Rcherche d'un point où la tangente ( T ' ) est paralelle ( T ) .

                 •  Considérons:      h '( x ) = -  1 / 4   avec x non nul

                      c-à-d               - 1 / x²   = - 1 / 4

                       c-à-d      x²  = 4   

                       c-à-d    x = 2   ou x = - 2

                    Considérons x = - 2  

                  •  Donnons l'équation réduite de la tangente à ( H ) au point d'abscisse - 2.

                   h '( - 2 ) = - 1/ 4      et     h( - 2 ) =- 1 / 2

               En reportant dans y = h '( - 2 ) x + h( - 2 ) - ( - 2 ) × h '( - 2)

              on a :    y = ( - 1 / 4 ) x  - ( 1 / 2 ) + 2 × ( - 1/ 4  )

             c-à-d      y = ( - 1 / 4 ) x - ( 1 / 2 ) -1 /2

              c-à-d      y = (- 1 / 4 )  x - 1

         Conclusion : L'équation  réduite de T ' est :     y = ( - 1 / 4 ) x - 1    

------------------------------------------------------------------------------------------------------------------------

       EXERCICE  2

                                   Soit la fonction rationnelle  f  :x  → ( 2 x + 1 ) / ( x – 2 ) 

                                   Donner  Df  , Dd   ,  f ' .

----------------------------------------------------------------------------------------------------------------------

   Réponse:        •   Df  = Dd   = IR- {2 }

                                En effet :

                              La fonction f est une fonction rationnelle définie

                              dans IR- { 2 }. Elle est donc dérivable sur son

                              domaine de définition.

                           •      f ’ :x  → - 5  / ( x – 2 )²

                                En effet :

                             f = u / v     avec     u : x →2 x + 1

                                                              v : x  → x - 2

                        Les fonctions u et v sont définies et dérivables

                        dans IR - { 2 }.   v est non nulle dans IR - { 2 }.

                        On a :                    u ' : x →  2           

                                                        v ' : x  → 1

                         On a :        f ' = ( u / v )' = ( v u ' - u v ' ) / v²

                          Soit x dans  R - { 2 }.

                           f '( x ) = ( ( x - 2 ) ×2 - ( 2 x + 1 ) × 1 ) / ( x - 2 )²   

         c-à-d        f '( x ) = ( 2 x - 4 - 2 x - 1 ) /    ( x - 2 )²     

         c-à-d          f '( x ) = - 5 /  ( x - 2 )²

------------------------------------------------------------------------------------------------------------

     EXERCICE 3

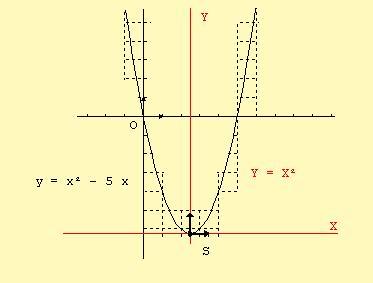
                   Soit la fonction polynôme  g : x →  x² - 5 x

                   Soit ( P ) sa courbe représentative dans un repère orthonormal ( O ; vect( i ) , vect( j ) ).

     1.        Tracer ( P ).

      1.  Le sommet  de la parabole ( P )  est :   S(    - b / ( 2 a); - Δ / ( 4 a) )

             c-à-d                     S( 2,5  ;  - 6,25 )

           En effet :    - b / ( 2 a) = 5 / 2        et        Δ = 25     Ainsi    - Δ / ( 4 a) = - 6,25 

           Dans le nouveau repère ( S:vect(i), vect(j ) ) , la parabole ( P) a pour

          équation Y = a X²   avec a = 1 .

             La parabole se trace donc très facilement.

             2. La tangente T au point d'abscisse 2 est :

                               .  T : y = - x - 4

                 En effet :

                      g ' : x  →  2 x - 5         

                  Ainsi :  g '( 2 ) = 4 - 5 = -1

                    g( 2 ) = 4 - 10 = - 6

                  En reportant dans  y = g '( 2) x + g ( 2 ) - g '( 2 ) × 2

                    il vient  :     y = - x - 6 - ( - 1 ) × 2

                       c-à-d        y = - x - 4

-----------------------------------------------------------------------------------------------------------------------------------

          EXERCICE 4

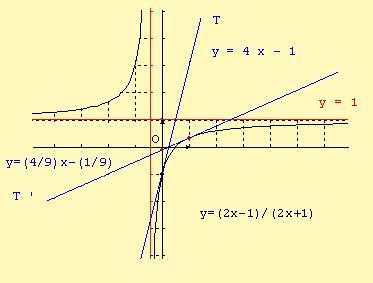
                                      Soit la fonction    h : x →  ( a x + b ) /  ( 2 x + 1)

               1. Peut-on trouver  deux réels   a et b  tels que ?

                      h( 0 ) = - 1       h( 1 ) = 1 / 3

                      h ‘ ( 0 ) = 4        h’ ( 1 ) = 4 / 9

               2. Tracer  alors la courbe ( C ) de h dans un repère orthonormal

                      ( O ; vect( i )  , vect( j ) )  ainsi que les tangentes aux points d’abscisse 0 et 1 .

------------------------------------------------------------------------------------------------------------------------------

      Réponse :

             1.    a = 2  et  b = - 1

                  En effet:

                   •  h( 0 ) = -1  s'écrit    b / 1 = - 1   c-à-d   b = - 1

                   •   h( 1 ) = 1 / 3  s'écrit   :       (  a + b  ) / 3 = 1 / 3   c-à-d   a + b = 1

                       comme b = - 1   il vient    a = - b + 1 = - ( - 1 ) + 1 = 2   c(à-d  a = 2

                  •   Calculons h ' ( x ) pour x distinct de - 0,5.

                       Soit x dans IR- { - 1/ 2 }  et h( x ) = (2 x -1 )  / (2 x + 1 )

                     On aobtient  facilement     h'( x ) = 4 / ( (2 x + 1 )²  

                     Donc h '( 0 ) = 4            et h ' ( 1 ) = 4 / 3²    = 4 / 9

                Les conditions sont bien respectées quand a = 2 et b = - 1

             Conclusion :   h : x  → ( 2 x -1 )  / (2 x + 1 )

              2. Courbe.      

---------------------------------------------------------------------------------------------------------------------------------

                                   Bon courage